§ 6: PM as a base for geometries with a finer structure

We start from the real projective plane of the straight lines and planes through O. A projective transformation is a bijection from this projective plane onto itself that preserves the incidence of points and lines and the cross ratio.
Let GP be the group of projective transformations, and LR³ the group of regular linear transformations of ℜ³. For A ∈ LR³, let φ_A be the projective transformation induced by A (see O61). Then A→φ_A is a surjective group homomorphism from LR³ to GP with kernel {λI / λ≠0}. So GP ≅ LR³/{λI / λ≠0}.
Let M³ be the group of regular 3 x 3 matrices with real coefficients, so that M³ ≅ LR³, and let E₃ be the 3 x 3 matrix with 1 on the diagonal and 0 off the diagonal. Then we also have GP ≅ M³/{λE₃ / λ≠0}. So, every projective transformation has a matrix that is unique up to a multiplication with a scalar λ.

Now take an arbitrary line out of P² and call it the line at infinity. We ourselves take the line l_∞ corresponding with {x₃=0}.
The projective transformations that map l_∞ onto itself form a subgroup of GP, the affine group GA, and are called affine transformations.
In this § we further identify each affine transformation with a regular linear transformation of ℜ³ that maps {x₃=0} to itself and (0,0,1) to (a,b,1). The matrix then has as its last row 0 0 1 and maps the points of {x₃=1} according to

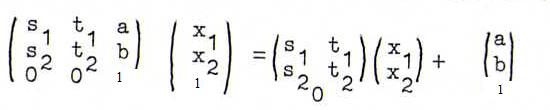
; hence, such an affine transformation is the product of: first an orthogonal projection onto {x₃=0}, then a linear transformation in {x₃=0}, and finally a translation with translation vector (a,b,1).

A similarity transformation is an affine transformation that works in {x₃=0} as a product of a rotation or reflection and a positive scalar (the similarity factor). So the matrix of a similarity transformation has the form

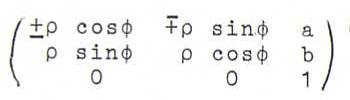
(with similarity factor ρ).
The similarity transformations form the similarity group GS.

Finally we define a congruence transformation as a similarity transformation with similarity factor 1. The congruence transformations form the congruence group GC.

Thus we find GC⊂GS⊂GA⊂GP.
For X∈{P, A, S, C} we define a X-concept as a concept that is invariant under the transformations of GX.
So {P-concepts}⊂{A-concepts}⊂{S-concepts}⊂{C-concepts}.
For example, points, lines and incidence are P-concepts, parallel is an A-concept, angle a S-concept, and distance a C-concept.

We define the X-geometry as the geometry of the X-concepts. The C-geometry is the good old euclidian geometry.

O22 Define the concept "parallel" in A-geometry, and show this is not a P-concept.

O23 Prove that "angle" is a S-concept, and "distance" a C-concept.

O24 Give for each of the following concepts the letters X so that that concept is a X-concept: quadrilateral, parallellogram, rhomb, square.

O25 We call two figures X-equal if there is an element of GX that maps one of them into the other. Are any two conics A-equal?

O26 Suppose we have three proper points A, B and C on a line l, and the point at infinity L_∞ on l.
Define the following A-concepts using cross ratio (We count ∞/∞=1; a/∞=0, ∞/a=∞ for a≠∞,0 (resp.); XY=-YX; etc.)
i) "X lies between A and B on l ";
ii) "AB is three times as great as BC ";
iii) "B is the centre of AC ".

answers